This formula is used to calculate the EOQ of a part, with the specific purpose of minimizing storage and ordering costs.
The formula is a theoretical aid that requires that certain parameters must have been established for the part. This applies to demand - in this case the annual volume; as well as the cost - in this case the standard price. Furthermore there must be an "ordering cost" established for the purchase or manufacturing, as well as a "holding cost" (also known as "carrying cost"). Based on these parameters, Wilson's formula can calculate the EOQ of a part using the following formula:
EOQ = (2KD/h)½
K = Ordering cost
D = Annual volume
h = Holding cost (which is the part's standard price x holding cost)
This means that the formula does not consider any intransit inventory, planning horizons, shortage costs or capital supply. Nor does it consider any quantity discounts or lower transport costs per unit when purchasing large quantities.
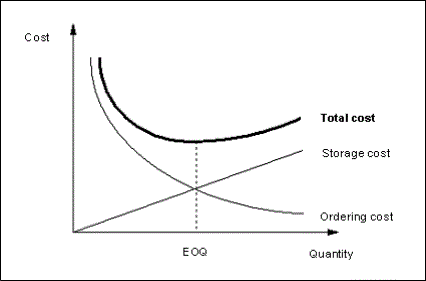
In order to see how the system calculates the EOQ using Wilson's formula, we have included a chart below. Here you can see how the storage cost increases and the ordering cost decreases as the quantity increases. The point where these two curves meet or cross one another is where the lowest total cost is. The quantity at this cost is therefore the part's most economic order quantity, EOQ.

Chart presentation of storage costs and ordering costs as a function of the order quantity.
Here is an example
The following conditions apply for a purchased product:
Standard price: 15:-
Annual volume: 2000 pcs
It costs the company 50:- in administration costs to place an order for this part = ordering cost.
The holding cost is estimated to be 5% of the product’s inventory value.
Wilson's formula will then be:
EOQ = (2x50x2000/(0,05x15))½ = 516 pcs.
If you also instructed the system to round-off the value to even 10's, the final EOQ = 520 pcs. Conclusion: If you purchase 520 pieces at a time, you will minimize the total cost for purchasing and warehousing this part.